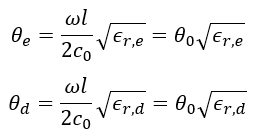Introduction
Coupled microstrip lines exhibit strong far-end crosstalk (FEXT) due to unequal phase velocities of the even and odd propagation modes. The diagram in Figure 1 shows that, in the case of the even-mode, a larger portion of E-field is travelling in the substrate material and therefore the effective dielectric constant






 Figure 2. Decomposition of the two-fold symmetrical four-port into four one-ports terminated by o.c. and s.c. within the symmetry planes.
Figure 2. Decomposition of the two-fold symmetrical four-port into four one-ports terminated by o.c. and s.c. within the symmetry planes.
Analysis of Compensated Coupled Microstrip Lines
Figure 1 shows two microstrip lines, which are coupled through the length





(1)
The characteristic impedances of the coupled transmission lines can be normalized as follows:
(2)
The normalized characteristic admittances of the even- and odd-mode can be calculated by inversion of the impedances:

The two-fold symmetrical four-port can be decomposed into four one-ports10,11, as depicted in Figure 2. These four one-ports are terminated by different combinations of o.c.and s.c. within the symmetry planes. Alternatively, o.c. and s.c. are often called magnetic and electric walls, respectively. The corresponding normalized eigenadmittances of the network can be calculated by transmission line theory:

(4-7)
The first and second index denote the mode with respect to symmetry plane A and B, respectively. The electrical length of the even and odd-mode is referred to as
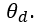
(8)
Scattering parameters can be easily calculated as they are linear combinations of the eigenreflections:
(9, 10, 11, 12)
 Figure 3. Typical constellation of eigenreflections (dashed: uncompensated, solid: compensated) in the admittance smith chart for a single frequency.
Figure 3. Typical constellation of eigenreflections (dashed: uncompensated, solid: compensated) in the admittance smith chart for a single frequency.
A typical constellation of eigenreflections for two coupled microstrip lines with
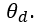





As the FEXT shall be minimized, the desired scattering parameter

(13)
These two criteria must be fulfilled at the same time in order to force
(14)
By inserting the Equations 4-7 into Equation 14 and solving for the capacitance value, the two competing results can be obtained:
(15, 16)
With the help of common trigonometric addition formulas, Equations 15 and 16 can be written as:
(17, 18)
It can be seen that
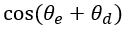
(19)
The electrical length

(20, 21)
with the angular frequency

(22)
Therefore, perfect isolation

(23)
When assuming that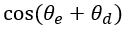
(24)
For
(25, 26)
Equation (25) presents the relation between the length of the coupled line structure and the frequency where perfect isolation can be achieved when compensation capacitances as calculated in (26) are used. For small arguments of the tangent-function, i.e. small differences between

(27)
This approximation may be sufficient in many cases, however, in the following the exact solution according to equation (25) and (26) is used. The example in the next section shows that improvement of isolation is not only obtained for the frequency
Example
Let’s assume an example with two microstrip lines of width 1.73 mm with 1 mm space between them on a Rogers RO4003C high frequency laminate, having a dielectric permittivity of 3.55 and a substrate height of 32 mils (which equals 0.813 mm). The length of the coupled section shall be 50 mm. The even and odd parameters are approximately





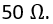


 Figure 4. Calculated S-parameters, uncompensated and compensated.
Figure 4. Calculated S-parameters, uncompensated and compensated.  Figure 5. Circuit topology with multiple compensation capacitors.
Figure 5. Circuit topology with multiple compensation capacitors.  Figure 6. Calculated S-parameters, uncompensated and compensated with multiple capacitors.
Figure 6. Calculated S-parameters, uncompensated and compensated with multiple capacitors.  Figure 7. Time-domain simulation results.
Figure 7. Time-domain simulation results. Conclusion
A method for the determination of capacitor values for compensation of unequal phase velocities in coupled microstrip lines has been presented. Simulation results show that broadband performance can be achieved with multiple capacitors along the coupled lines. In practice, the low capacitance values (a couple of femto-Farads) can be realized by stubs, interdigital capacitors, or the modulation of the space between adjacent microstrip lines. The presented equations help to find the maximum allowed distance between the capacitive elements and their required optimum capacitance values. In reality, there are, of course, often more than two transmission lines. Losses have to be considered, as perfect symmetry is not a given and other non-idealities have to be taken into account.
References
1. R. K. Kunze, Y. Chu, Z. Yu, S. K. Chhay, M. Lai, and Y. Zhu, “Crosstalk mitigation and impedance management using tabbed lines,” Intel White Paper, 2015.
2. Wu, C.H., Shen, L., Zhang, H. et al. Equivalent circuit parameters of planar transmission lines with spoof surface plasmon polaritons and its application in high density circuits. Sci Rep 9, 18853 (2019).
3. Q. -M. Cai, Y. Zhu, R. Zhang, Y. Ren, X. Ye and J. Fan, "A Study of Coverlay Coated Microstrip Lines for Crosstalk Reduction in DDR5," 2020 IEEE International Symposium on Electromagnetic Compatibility & Signal/Power Integrity (EMCSI), Reno, NV, USA, 2020, pp. 581-585.
4. A. Podell, "A high-directivity microstrip coupler technique," 1970 IEEE MTT-S Int. Microwave Symp. Dig., pp. 33-36, 1970.
5. G. Haupt and H. Delfs, “High-directivity microstrip directional couplers,” Electron. Lett., vol. 10, pp. 142–143, May 1974.
6. Broadband directional couplers using microstrip with dielectric overlays," IEEE Trans. Microwave Theory and Tech., vol. MTT-22, pp. 1216-1220, Dec. 1974.
7. G. Schaller, “Optimization of microstrip directional couplers with lumped capacitors,” Int. J. Electron. Commun. (AEÜ), vol. 31, pp. 301–307, 1977.
8. M. Dydyk, “Accurate design of microstrip directional couplers with capacitive compensation,“ IEEE MTT-S Symp. Dig., pp.581-584, 1990.
9. C. T. Nghe, F. Rautschke and G. Boeck, "Performance optimization of capacitively compensated directional couplers," 2017 47th European Microwave Conference (EuMC), Nuremberg, Germany, 2017, pp. 408-411.
10. C. G. Montgomery, R. H. Dicke, and E. M. Purcell, Principles of Microwave Circuits. New York, NY, USA: McGraw-Hill, 1948.
11. H. Mextorf and R. Knöchel, "The Intrinsic Impedance and Its Application to Backward and Forward Coupled-Line Couplers," in IEEE Transactions on Microwave Theory and Techniques, vol. 62, no. 2, pp. 224-233, Feb. 2014.